Question

Find the standard deviation of a series of ACT scores listed below.14, 16, 13, 31, 21The standard deviation is(Type an integer or decimal rounded to the nearest hundredth as needed.)

Answer 1

s = 7.38

Step-by-step explanation

The standard deviation, s, of a sample is the square root of the variance, s²,

`s^2=(1)/(n-1)\sum_{i\mathop{=}1}^n(x_i-\bar{x})^2`

Where n is the number of data, 5, and x with the line on top is the mean,

`\bar{x}=(14+16+13+31+21)/(5)=19`

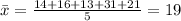
So the variance is,

`s^2=(1)/(5-1)((14-19)^2+(16-19)^2+(13-19)^2+(31-19)^2+(21-19)^2)`

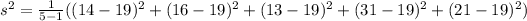
Solve the subtractions,

`s^2=(1)/(4)((-5)^2+(-3)^2+(-6)^2+(12)^2+(2)^2)`

Solve the squares, add and divide by 4,

`s^2=(1)/(4)(25+9+36+144+4)=(218)/(4)=54.5`

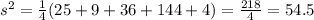
Finally, to find the standard deviation, take the square root,

`s=√(54.5)\approx7.38`

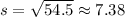
Hence, the standard deviation is 7.38, rounded to the nearest hundredth.