Question

Given:Point O is the center of three different circles shown below.ON = NL = LKKP = 42 inPINILKWhich is closest to the area of the shaded region?231 sq in.308 sq in.539 sq in.616 sq in.

Answer 1

line KP is the Diameter = 42

radius total = radius of the largest circle = diameter/2 = 42/2 = 21,

ON = NL = LK ........given from the question....we can represent this as x

that is,

x + x + x = radius total

3x = 21,

x = 21/3 = 7

That is, ON = NL = LK = 7

Now, radius small = radius of the smallest circle = ON = 7.

Let's calculate the Area of the largest circle...........

Area total = pi * radius total

`\begin{gathered} A_T=pi* r_T^2 \\ A_T\text{ = 3.142 }*21^2\text{ = 1385.622 sq in} \end{gathered}`

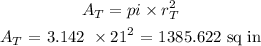
Let's calculate the Area of the smallest circle...........

Area small = pi * radius small

`\begin{gathered} A_S\text{ = pi }* r_S^2_{} \\ A^{_{}}_S\text{ = 3.142 }*7^2\text{ = 153.985 sq in} \end{gathered}`

Now , to calculate the area of the shaded region, we have to substract the area of the smallest from the largest and divide the answer by 2

Area shaded = (1385.622 - 153.985) / 2 = 1231.664 / 2 = 615.832 = 616 sq in

That's all

Thank you!