We are given the following information.
Person 1 rode the subway three times at the peak fare price and five times at the off-peak fare price for a total cost of $19.50.
Person 2 rode two times at the peak fare price and four times at the off-peak fare price for a total cost of $14.50.
We are asked to find the peak fare price.
Let x represent the peak fare price.
Let y represent the off-peak fare price.
Then we can set up the following two equations for persona 1 and person 2.
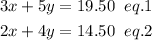
Separate out the y variable in eq.1
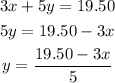
Now, substitute it into eq.2
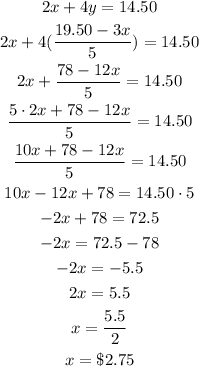
Therefore, the peak fare price is $2.75