Question

Ok I’m sorry but I didn’t do one

Answer 1

Given the graph shows the function:

y(x)

Let's use the graph to estimate y'(a) at different locations.

Using the given graph, we can see the line shows a negative slope.

Apply the slope-intercept form equation with a negative slope:

`y=-mx+b`

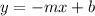
Where:

m and b represent the slope and y-intercepts respectively which are constants.

Now, to find y', let's find the derivative d/dx:

`\begin{gathered} (dy)/(dx)=-mx+b \\ \\ y^(\prime)=(dy)/(dx)(-mx)+(dy)/(dx)(b) \\ \\ y^(\prime)=-m \end{gathered}`

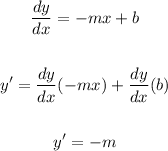
This means the line will be a horizontal line on the negative side of the y-axis.

Therefore, the graph which shows y'(x) is:

ANSWER: