Question

Graph the parabola.y=x2-4Plot five points on the parabola: the vertex, two points to the left of the vertex, and two points to the right of the vertex. Then click on the graph-a-functionbuttonХ5?

Answer 1

Given the Quadratic Function:

`y=x^2-4`

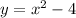
You need to remember that the Quadratic Parent Function (the simplest form of a Quadratic Functions) is:

`y=x^2`

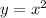
And its vertex is at the Origin:

`(0,0)`

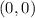
The function given in the exercise was obtained by shifting the parent function 4 units down. That means that the y-coordinate of the vertex changes, but the x-coordinate is the same. Therefore, the vertex of this parabola is:

`(0,-4)`

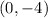
To find two points to the left of the vertex, you can choose this value for "x":

`x=-1`

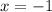
Substituting this value into the function and evaluating, you can find the corresponding value of "y":

`y=(-1)^2-4=1-4=-3`

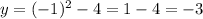
Now you have this point:

`(-1,-3)`

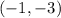
You can choose this value of "x":

`x=-4`

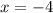
And apply the same procedure:

`y=(-4)^2-4=16-4=12`

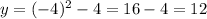
Then, the other point is:

`(-4,12)`

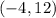
To find the first point to the right of the vertex, you can substitute this value of "x" into the function and evaluate:

`x=1`

Then, you get:

`y=(1)^2-4=-3`

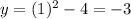
The point is:

`(1,-3)`

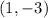
To find the second point, substitute this value into the equation and evaluate:

`x=4`

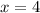
Then:

`y=(4)^2-4=16-4=12`

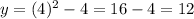
So the other point is:

`(4,12)`

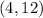
Now you can plot the points and graph the function.

Therefore, the answer is: