1)
In order to find the velocity, we just need to divide a distance by the corresponding time.
So, using the point (2, 120), that is, a distance of 120 for a time of 2, we have:
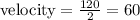
2)
Is it a function? Yes, since each value in the x-axis has only one corresponding value in the y-axis.
3)
To find the rule of this function, we can use the slope-intercept of the linear function (y = mx + b) and two points of the graph, for example (0, 0) and (2, 120), so we have:
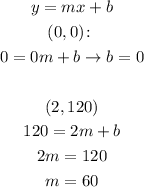
So our function is y = 60x
4)
Using the rule we found, we can write the table: