Question

Given function f(x) = 2x^5 + 5x^4, use first derivative test to determine:A) minimum and maximum points on the interval [-2,1]B) intervals of increase and decrease on interval (-infinity,infinity)

Answer 1

We need to find the maximum and minimum points on [-2,1] for the function

`f(x)=2x⁵+5x⁴`

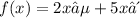
Then

`f^(\prime)(x)=10x⁴+20x³`

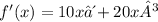
Now to find the critical points we have that

`f^(\prime)(x)=0\leftrightarrow10x⁴+20x³=0`

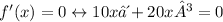
so

`10x⁴+20x³=10x³(x+2)=0\text{ }\leftrightarrow x=0\text{ or }x=-2`

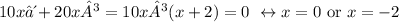
We get that we have two critical points at the interval [-2,1].

Now, to determine if they are minimum or maximum points we choose any point before and after them. We will choose -3,-1,1.

So,

`f^(\prime)(-3)=10(-3)⁴+20(-3)³=10(81)-20(27)=810-540>0`
`f^(\prime)(-1)=10(-1)⁴+20(-1)³=10-20=-10<0`
`f^(\prime)(1)=20(1)³+10(1)⁴=30>0`

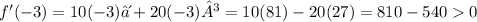
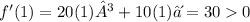
We should notice that f'(-3)>0 f'(-2)=0 and f'(-1)<0. Then

`x=-2\text{ is a maximum point}`

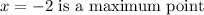
Moreover, since f'(-1)<0 f(0)=0, f'(1)>0. Then

`x=0\text{ is a minimum point }`

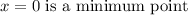
(a) We have that x=-2 is a maximum point and x=0 is a minimum point on the interval [-2,1]

To find the intervals where the function increase and decrease, notice that

`f^(\prime)(x)>0,\text{ for }x\in(-\infty,-2).\text{ Then }f\text{ increases on }(-\infty,-2).`

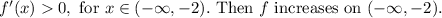
since f'(x) is a continuous polynomial and f'(-2)=0 and f'(-3)>0.

Now,

`f(x)\text{ decreases on }(-2,0)`

since f'(-2)=0 and here attaches a maximum, then the function needs to decrease until the nex critical point that is the point x=0.

After taking the minimum point x=0, f(x) will increase, i.e.

`f(x)\text{ increases on }(0,\infty)`

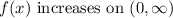
(b) f(x) increases on (-infinity,-2), decreases on (-2,0) and increases once more time on (0,infinity)

`f(x)=0\leftrightarrow x⁴(2x+5)=0\leftrightarrow x=0\text{ or }x=(-5)/(2)`

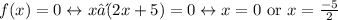
So,