1 Answer

Margherita · Answer 1 · 2023-12-28T02:36:06+0000

First, we have to find the acceleration of the car with the following formula.

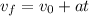
Where vf = 0, v0 = 84.21 km/h, and t = 9.260 s. Let's use these values and solve for a.

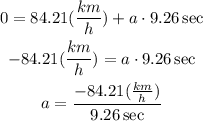
$\begin{gathered} 0=84.21((km)/(h))+a\cdot9.26\sec \\ -84.21((km)/(h))=a\cdot9.26\sec \\ a=(-84.21((km)/(h)))/(9.26\sec ) \end{gathered}$

Before we divide, we have to express the speed in meters per second. We know that 1 km equals 1000 meters, and 1 hour equals 3600 seconds.

$\frac{84.21\operatorname{km}}{h}\cdot\frac{1000m}{1\operatorname{km}}\cdot(1h)/(3600\sec )\approx23.39((m)/(s))$

Then, we use the transformed speed to find the acceleration.

$a=(-23.39((m)/(s)))/(9.26\sec )\approx-2.53((m)/(s^2))$

Once we have the acceleration, we can find the net force using Newton's Second Law.

Where m = 1,052 kg and a = - 2.53 m/s2.

$F=1052\operatorname{kg}\cdot(-2.53((m)/(s^2)))=-2661.56N$

Therefore, the net force is 2661.56 Newtons.

The negative sign indicates that the force applied is developing a negative acceleration on the car.