Answer:
297 degrees
Step-by-step explanation:
Given the vector: w=4i-8j
The x-coordinate is positive while the y-coordinate is negative, this implies that w is in Quadrant IV.
First, we find α below:

Next, we find the direction angle below:
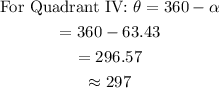
The angle that w makes with the positive x-axis (measured counterclockwise) is 297 degrees.