Question

I am unsure where to start with number 3 and it is a little confusing

Answer 1

When we have two roots of the same kind being multiplied, we can put them together, like this:

`\sqrt[3]{2x^5y^5}\cdot\sqrt[3]{16x^3y^7}=\sqrt[3]{2x^5y^5\cdot16x^3y^7}`

Now, we can reorder the factors so that we pair the numbers, the x variable and the y variables:

The 16 we can write as its factors, and the variables we can put together using:

`b^a\cdot b^c=b^(a+c)`

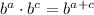
Doing so, we have:

`\sqrt[3]{2\cdot2^4\cdot x^(5+3)\cdot y^(5+7)}=\sqrt[3]{2^5x^8y^(12)}`

Now, since the root is cubic root, for every 3 repetitions of a factor, we cancel it with the root.

So, for instance, we have 5 factors 2, so if we pick three of them, we can cancel with the cuibc root. We can do this to x and y too:

`\sqrt[3]{2^32^2x^3x^3x^2y^3y^3y^3y^3}=\sqrt[3]{2^3}\sqrt[3]{x^3}\sqrt[3]{x^3}\sqrt[3]{y^3}\sqrt[3]{y^3}\sqrt[3]{y^3}\sqrt[3]{y^3}\sqrt[3]{2^2x^2}=2\cdot x\cdot x\cdot y\cdot y\cdot y\cdot y\sqrt[3]{4x^2}=2x^2y^4\sqrt[3]{4x^2}`

So, the simplification becomes:

`\sqrt[3]{2x^5y^5}\cdot\sqrt[3]{16x^3y^7}=2x^2y^4\sqrt[3]{4x^2}`