Question

A restaurant owner was experimenting with the taste of her home style Leland drink. She started with 60 liters of water. She removed 15 liters of the water and replaced it with 15 liters of pure Komondor juice. After thoroughly stirring the new mixture, she discovered it was to lemony. So she she removed 10 liters of the new mixture and replaced it with 10 liters of water. She thoroughly stirred the mixture and concluded that the new mixture was just right. Determine the ratio of pure lemon juice to water in the final 60 liters of mixture.

Answer 1

First she have 60 liters of water, then she remove 15 liters of water and replace it with 15 liters of lemonade, so we can calculate the ratio of this first mix.

`(45)/(15)=3`

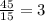
This means that for each 3 liters of water we have one liter of lemonade, so in each liter of the mixture we have 3/4 of water fo 1/4 of lemonade so in ten liters will be:

`\begin{gathered} (3)/(4)10=(30)/(4)=(15)/(2)=7.5 \\ (1)/(4)10=(10)/(4)=(5)/(2)=2.5 \end{gathered}`

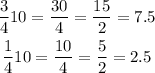
this means that in in the mixture are 37.5 liters of water, and 12.5 liters of lemonade, then she put 10 liters of water so, now there are 47.5 liters of water and 12.5 liters of lemonade, so we can calculate the final ratio:

`(47.5)/(12.5)=3.8`

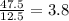
this means that for each liters of lemonade there are 3.8 liters of water