We are given the following quadratic equation.
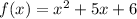
Recall that the standard form of a quadratic equation is given by

Comparing the given equation with the standard form, the coefficients are
a = 1
b = 5
c = 6
The vertex point is given by
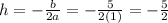
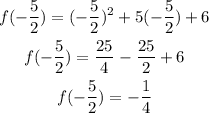
So, the vertex point is
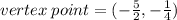
Now let us find the y-intercept of this equation.
Substitute x = 0 into the equation
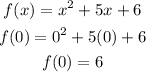
So, the y-intercept is
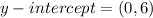
Finally, let us find the x-intercept of this equation
Substitute f(x) = 0 into the equation
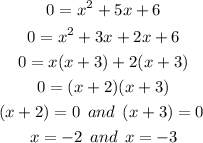
So, the x-intercepts are
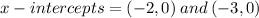
Conclusion:
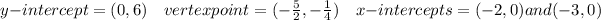
Therefore, the correct answer is option B