Question

The height y (in feet) of a ball thrown by a child is:Y= -1/12x^2 +4x+5.Where x is the horizontal distance in feet from the point at which the ball is thrown.A. How high is the ball when it leaves the child’s hand?B. What is the maximum height of the ball?C. How far from the child does the ball strike the ground?

Answer 1

`y=-(1)/(12)x^2+4x+5`

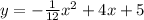
when the ball leaves the chil'd hand the distance is 0

so:

`\begin{gathered} y=(-(0)^2)/(12)+4(0)+5 \\ y=\text{ 0+0+5} \\ y=5 \end{gathered}`

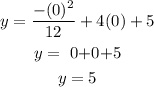
the height is 5 feets

The maximun height happens when x=-b/(2a) because is the vertex

where b=4 and a=-1/12

`\begin{gathered} x=(-b)/(2a) \\ \\ x=(-(4))/(2((-1)/(12))) \\ \\ x=(-4)/(-(1)/(6))\text{ } \\ x=24 \end{gathered}`

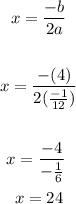
so x is the distance where maximun height happens, so replace on the function

`\begin{gathered} y=-(1)/(12)x^2+4x+5 \\ \\ y=-(1)/(12)(24)^2+4(24)+5 \\ y=-(1)/(12)(576)+96+5 \\ y=-48+96+5 \\ y=53 \end{gathered}`

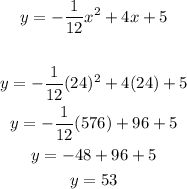
the maximun height is 53 feets

the ball strike the ground when the height is 0 so we can replace y and fin x or the distance

`\begin{gathered} 0=-(1)/(12)x^2+4x+5 \\ \end{gathered}`

we need to factor and we can do it with this equation

`x=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a}`

where a= -1/12 , b=4 and c=5

`\begin{gathered} x=\frac{-(4)\pm\sqrt[]{(4)^2-4(-(1)/(12))(5)}}{2(-(1)/(12))} \\ \\ x=\frac{-4\pm\sqrt[]{16+((5)/(3))}}{-(1)/(6)} \\ x=-6(-4\pm\sqrt[]{(53)/(3)}) \\ \\ x=-6(-4\pm4.20) \end{gathered}`

we have 2 solutions

1.

`\begin{gathered} x_1=-6(-4+4.20) \\ x_1=-6(0.2) \\ x_1=-1.2 \end{gathered}`

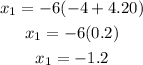
but its not a real solution because distance cant be negative

2.

`\begin{gathered} x_2=-6(-4-4.20) \\ x_2=-6(-8.2) \\ x_2=49.2 \end{gathered}`

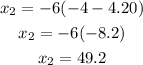
so the distance when the ball strike the ground is 49.2 feets