Hello!
First, we have to remember that the equation of the line has the form:
y = mx + b
• m, = slope
,
• b, = y-intersection
Knowing it, we can solve this exercise:
1st equation: 5x -6y = 30
Let's isolate y:
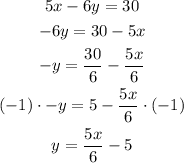
Slope: 5/6
Y-intercept: (0, -5)
2nd equation: y = 5 -6x
Slope: -6x
Y-intercept: (0, 5)
3rd equation: y = 5/6x +1
Slope: 5/6
Y-intercept: (0, 1)
4th equation: 5x -6y = 6
Let's isolate y:

Slope: 5/6
Y-intercept: (0, -1)
5th equation: 5x + 6y = 6
Let's isolate y:
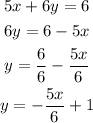
Slope: -5/6
Y-intercept: (0, 1)
6th equation: 6x +y = 12
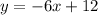
Slope: -6
Y-intercept: (0, 12)
Look at your answers below: