Question

Solve zero equals X squared minus 7X -18

Answer 1

We must solve for x the following equation: "zero equals X squared minus 7X -18", mathematically this equation is:

`0=X^2-7X-18.`

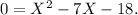
Solving this equation is equivalent to find the roots of a polynomial of degree 2. A polynomial of degree 2 always has 2 roots, and they are given by the following equations:

`\begin{gathered} x_1=\frac{-b+\sqrt[]{b^2-4ac}}{2a}, \\ x_2=\frac{-b-\sqrt[]{b^2-4ac}}{2a}, \end{gathered}`

where the coefficients a, b and c are coefficients of each term of the polynomial:

`aX^2+bX+c\text{.}`

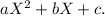
Comparing with the polynomial of the problem, we see that the coefficients are:

`a=1,b=-7,c=-18.`

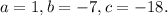
Replacing the values of the coefficients in the formulas for the roots above, and computing, we get:

`\begin{gathered} x_1=\frac{-(-7)+\sqrt[]{(-7)^2-4\cdot1\cdot(-18)}}{2\cdot1}=9, \\ x_2=\frac{-(-7)-\sqrt[]{(-7)^2-4\cdot1\cdot(-18)}}{2\cdot1}=-2, \end{gathered}`

Answer

The solutions to the equation of the problem are:

`\begin{gathered} x_1=9, \\ x_2=-2. \end{gathered}`