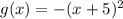The first thing we notice is that the function is reflected. So we can start by reflecting it with respect to the x-axis.
We do this by adding a negative sign in the function:

Now, we have the function reflected, but in the wrong position. We can track its position by the vertex. It was originally at (0,0) and remains at (0,0) after the reflection.
But the final function have its vertex at (-5,0), so we have to translate the function 5 units to the left. we do this by adding 5 to the x in the function:
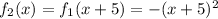
Now, to check if there isn't any dilatation, we can check on other point in the graph to see if it checks out.
In the blue graph, we see the point (-3,-4), so let's input x = -3 and see if it checks out:
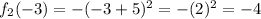
We got y = -4, so it checks out.
Thus, the answer is: