We are given the surface area of a cone and we are asked to find its diameter. To do that, let's remember the formula for the surface area of a cone:
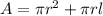
Where "r" is the radius, "h" is the height and "l" is the slant height. We are given the following values:
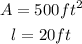
Replacing in the formula we get:
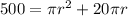
Now we need to solve for "r", to do that we will subtract 500 on both sides:
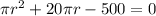
Now we will use the quadratic formula to find the values of "r". The quadratic formula is the following:
![r=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a}](https://img.qammunity.org/2023/formulas/mathematics/college/tw1mhsr6g1g4rwd5o7rle7wu167ecb7ewa.png)
Where:
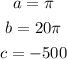
Replacing in the quadratic formula we get:
![r=\frac{-20\pi\pm\sqrt[]{400\pi^2-4(\pi)(-500)}}{2\pi}](https://img.qammunity.org/2023/formulas/mathematics/college/123dxrn4scwiw57ob1e5j6fi8ayza1g6wo.png)
simplifying:
![r=\frac{-20\pi\pm\sqrt[]{400\pi^2+2000\pi}}{2\pi}](https://img.qammunity.org/2023/formulas/mathematics/college/du8j8x98a6sxtpcx4pwrkybwf24ci22f8o.png)
Solving the operations inside the radical we get:

Now we take the positive sing and solve the operations, like this:
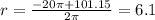
Taking the negative sing we get:
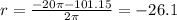
Since the radius should be a positive quantity we take the first value, therefore the radius is 6.1 ft. To get the diameter we use:
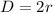
Therefore the diameter is:
