Question

What is the probability of winning the minimum award is

Answer 1

Given:

• Top cash prize = $697

,

• First set of numbers to pick 4 different numbers from = 1 to 53

,

• Second set to pick one number from = 1 to 46

,

• Minimum award = $225

Given that the player wins the minimum award by matching three numbers drawn from 1 to 53 and matching number through 1 to 43, let's find the probability of winning the minimum award.

To find the probability, we have the following:

Number of ways to pick 4 different numbers from 1 to 53:

`\begin{gathered} ^(53)C_4=(53!)/(4!(53-4)!)=(53!)/(4!*49!) \\ \\ ^(53)C_4=(53*52*51*50*49!)/(4!*49!)=(53*52*51*50)/(4*3*2*1)=(7027800)/(24)=292825 \end{gathered}`

Now, the probability of matching 3 numbers from the 4 different numbers picked and matching the number on the gold ball (1 to 46) will be:

`\begin{gathered} P=(^4C_3*(53-4))/(^(53)C_4)*(1)/(46) \\ \\ P=((4!)/(3!(4-3)!)*49)/(292825)*(1)/(46) \\ \\ P=((4*3!)/(3!*1!)*49)/(292825)*(1)/(46) \\ \\ P=(4*49)/(292825)*(1)/(46) \\ \\ P=(196*1)/(292825*46) \\ \\ P=(196)/(13469950) \\ \\ P=(98)/(6734975) \end{gathered}`

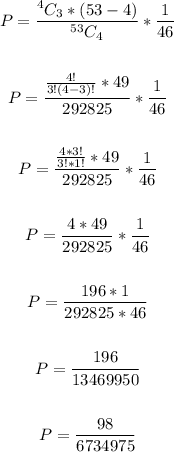
Therefore, the probability of winning a minimum award is:

`(98)/(6734975)`

• ANSWER:

`(98)/(6734975)`