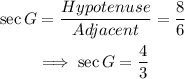Question

I need help with this practice problem solving Not sure how to solve step by step I believe the subject for this is trigonometry

Answer 1

`\begin{gathered} (a)\cot G=(3√(7))/(7) \\ (b)\sin E=(3)/(4) \\ (c)\sec G=(4)/(3) \end{gathered}`

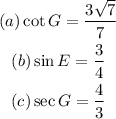
Explanation:

A sketch of the triangle and the given dimensions is attached below:

First, find the length of EF using the Pythagorean Theorem:

`\begin{gathered} EG^2=EF^2+FG^2 \\ 8^2=EF^2+6^2 \\ EF^2=8^2-6^2=64-36=28 \\ EF=√(28)=2√(7) \end{gathered}`

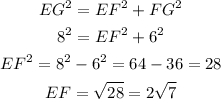
(a)cot G

Cotangent is the inverse of tangent.

• The side length opposite to G = 2√7

,

• The side length adjacent to G = 6

`\begin{gathered} \cot G=(Adjacent)/(Opposite)=(6)/(2√(7))=(3)/(√(7)) \\ Rationalising: \\ \cot G=(3)/(√(7))*(√(7))/(√(7)) \\ \implies\cot G=(3√(7))/(7) \end{gathered}`

(b)sin E

• The side length ,opposite, to E = 6

,

• The length of the ,hypotenuse, = 8

`\begin{gathered} \sin E=(Opposite)/(Hypotenuse)=(6)/(8) \\ \implies\sin E=(3)/(4) \end{gathered}`

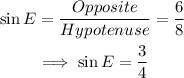
(c)sec G

Secant is the inverse of cosine.

• The side length adjacent to G = 6

,

• The length of the hypotenuse = 8

`\begin{gathered} \sec G=(Hypotenuse)/(Adjacent)=(8)/(6) \\ \implies\sec G=(4)/(3) \end{gathered}`