First, we'll convert the angle into degrees. We'll do so by replacing pi by 180, as following:
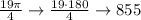
This way, we'll get that the angle is 855°. To this angle, we'll substract the nearest factor of 360: 720
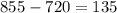
This way, we get an angle we can work on (between 0° and 360°)
Now, notice that 135° is an angle that belongs to the second quadrant. Because of this, we'll habe to substract 90° to get the reference angle:
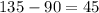
We get that the reference angle is 45°. Now, let's switch this angle back into radians. To do so, we multiplty by pi and divide by 180, as following:
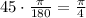
This way, we'll have that the reference angle for
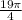
is:

And since cosine is negative in the second quadrant (where the original angle belongs), we can conclude that:
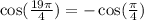
And we'll have that:
![-\cos ((\pi)/(4))=-\frac{\sqrt[]{2}}{2}](https://img.qammunity.org/2023/formulas/mathematics/college/ep7fiw6adw0x59tr4f0bpyzhrs40m7wvf4.png)