Answer:
Explanation:
We first solve for y in the second equation.
The second equation is
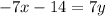
dividing both sides by 7 gives
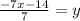
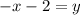
We now substitute the value of y into the first equation.
The first equation is
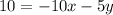
substituting the value of y gives

Simplifying the right-hand side gives

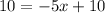
Subtracting 10 from both sides gives
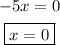
With the value of x in hand, we now put it into -7x - 14 = 7y to get

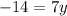
Dividing both sides by 7 gives

Hence, to conclude, the solution to the system is
x = 0
y = -2.
Therefore, x + y = -2