Let's first write both numbers in their fraction form:
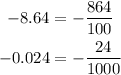
then, we can write the division like this:

Now, given the general rule for the division of fractions, we have:
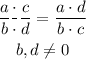
also notice that we are dividing two negative numbers, then our final result will be positive according to the law of signs. Then, we have in this case:

therefore the final result is 360