68 68 70 61 67 71 63 67
Step 1: Write the formula of standard deviation
![\text{Stanadard deviation = }\sqrt[]{\frac{Sum(x\text{ - }\mu)^2}{n}}](https://img.qammunity.org/2023/formulas/mathematics/high-school/yn82dwhwzwussjacobvn9w71vsp7pxpdsv.png)
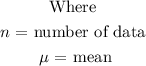
n = 8
Step 2: Find the mean
![\begin{gathered} \operatorname{mean}\text{ }\mu\text{ = }\frac{\sum ^{}_{\text{ }}x}{n} \\ \mu\text{ = }\frac{68\text{ + 86 + 70 + 61 + 67 + 71 + 63 + 67}}{8} \\ \mu\text{ = }(535)/(8) \\ \mu\text{ = 66.9} \end{gathered}]()
Step 3: find the standard deviation
Next, substitute to find the standard deviation
![\begin{gathered} \text{standard deviation = }\sqrt[]{\frac{sum(x\text{ - }\mu)^2}{n}} \\ =\text{ }\sqrt[]{(78.88)/(8)} \\ =\text{ }\sqrt[]{9.86} \\ =\text{ 3.14} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/high-school/v5hdbj6ul57mgc01xtu5ehb5rnfv50juvd.png)
standard deviation = 3.14
Final answer
The number of data within the standard deviation of the mean = 5