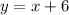Question

Write an equation in slope-intercept form of a line passingthrough the given point and perpendicular to the given line.Write an equation in slope-intercept forrthrough the given point and perpendicular ~ (-5,1); y= -X

Answer 1

The formula to find the equation of a straight line is

`m=(y-y_1)/(x-x_1)`

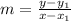
Given that the line passes through the point (-5,1) which is parallel to the given line

`y=-x`

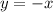
Since the lines are perpendicular, then to find the slope of the other line, the formula is

`m_2=-(1)/(m_1)`

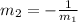
Let m₁ be the slope of the given line and m₂ be the slope of the line perpendicular to the given line

The general form of an equation of a straight line is

`\begin{gathered} y=mx+b \\ \text{Where } \\ m\text{ is the slope and b is the y-intercept} \end{gathered}`

The slope, m₁, of the given line is -1, i.e m₁ = -1

The slope, m₂, of the line perpedicular to the given line will be

`\begin{gathered} m_1=-1 \\ m_2=-(1)/(m_1) \\ m_2=-(1)/(-1) \\ m_2=1 \end{gathered}`

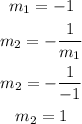
The slope, m₂, of the line passing through the points (-5, 1), m₂ = 1

Where

`\begin{gathered} (x_1,y_1)\Rightarrow(-5,1) \\ m_2=1 \end{gathered}`

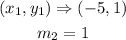
Substitute the variables into the formula to find the equation of a straight line

`\begin{gathered} m=(y-y_1)/(x-x_1) \\ 1=(y-1)/(x-(-5)) \\ 1=(y-1)/(x+5) \\ \text{Crossmultiply} \\ x+5=y-1 \\ y=x+5+1 \\ y=x+6 \end{gathered}`

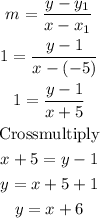
Hence, the equation of the line in slope-intercept form is

`y=x+6`