Question

How many distinct proper subsets are there of the set N = {8, 50, 69, 17, 33, 55, 28)

Answer 1

The number of proper subset of a set N is given as;

`\begin{gathered} P(N)=2^n \\ \text{Where n is the cardinality of the set} \end{gathered}`

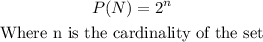
The cardinality of a set is the number of elements in a set.

Then, given the set;

`N=\mleft\lbrace8,\text{ 50, 69, 17, 33, 55, 28}\mright\rbrace`

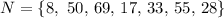
The cardinality n, is7 because there are seven elements in the set.

Then, the number of proper subsets is;

`\begin{gathered} P(N)=2^7 \\ P(N)=128 \end{gathered}`

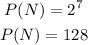
Thus, the set N has 128 distinct proper subsets.