Question

Determine the x-intercepts of the quadratic function f(x)= 2x^2 - 6x + 1give exact answers

Answer 1

Given the quadratic equation:

`2x^2-6x+1`

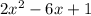
The x-intercepts are the values of (x) which make the equation equal to zero

Or, the x-intercepts are the values of intersection with the x-axis

So, we will equate the equation to zero then solve it for (x):

`\begin{gathered} 2x^2-6x+1=0 \\ \end{gathered}`

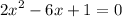
As we will not be able to factor the equation, we will use the general formula:

`x=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a}`

From the equation:

`a=2,b=-6,c=1`

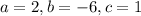
substitute with the values of {a, b, c} into the general formula:

`\begin{gathered} x=\frac{6\pm\sqrt[]{(-6)^2-4\cdot2\cdot1}}{2\cdot2}=\frac{6\pm\sqrt[]{28}}{4} \\ \\ x=\frac{6+\sqrt[]{28}}{4}=\frac{6+2\sqrt[]{7}}{4}=(3)/(2)+(1)/(2)\sqrt[]{7} \\ or \\ x=\frac{6-\sqrt[]{28}}{4}=\frac{6-2\sqrt[]{7}}{4}=(3)/(2)-(1)/(2)\sqrt[]{7} \end{gathered}`

So, the x-intercepts are:

`x=\mleft\lbrace(3)/(2)-(1)/(2)\sqrt[]{7},(3)/(2)+(1)/(2)\sqrt[]{7}\mright\rbrace`