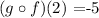Question

For the pair of functions, f(x) = x + 4 and g(x) = 4x - 3, find the following.a) (fog)(x)b) (fog)(2)c) (gof)(x)d) (gof)(2)a) (fog)(x) = 4x + 1 (Simplify your answer.)b) (fog)(2)= (Simplify your answer.)ingotscessessLibrary

Answer 1

Question:

Solution:

Consider the following functions:

`f(x)\text{ = x+4}`
`g(x)\text{ = }4x-3`

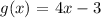
then:

`(f\circ g)(x)\text{ = f(g(x))= (4x-3)+4}`

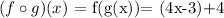
this is equivalent to:

`(f\circ g)(x)\text{ =(4x-3)+4 = 4x +1}`

thus:

`(f\circ g)(x)\text{ =4x +1}`

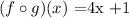
So, replacing x = 2 in the previous function we get:

`(f\circ g)(2)\text{ =4(2) +1 = 9}`

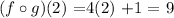
then:

`(f\circ g)(2)\text{ = 9}`

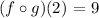
On the other hand, the composition:

`(g\circ f)(x)\text{ = g(f(x)) = 4(x+4)-3}`

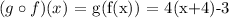
this is equivalent to:

`(g\circ f)(x)\text{ = 4(x+4)-3 = 4x +16-3 = 4x - 13}`

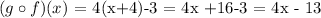
then, we can conclude that:

`(g\circ f)(x)\text{ =4x - 13}`

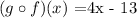
So, replacing x = 2 in the previous function we get:

`(g\circ f)(2)\text{ =4(2) - 13 = 8-13 = -5}`

then:

`(g\circ f)(2)\text{ =4(2) - 13 = 8-13 = -5}`

then:

`(g\circ f)(2)\text{ =-5}`

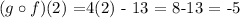
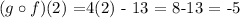
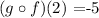
Then the correct answers are:

1)

`(f\circ g)(x)\text{ =4x +1}`

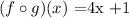
2)

`(f\circ g)(2)\text{ = 9}`

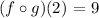
3)

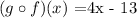
`(g\circ f)(x)\text{ =4x - 13}`

4)

`(g\circ f)(2)\text{ =-5}`