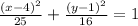Question

Write an equation for the given ellipse that satisfied the following conditions.

Answer 1

Given:

Center ==> (4, 1)

Length = 8

c = 3

Let's find the equation for the given ellipse that statisfies the conditions above.

Take the equation:

`((x-x1)^2)/(a^2)+((y-y1)^2)/(b^2)=1`

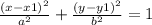
Here, we have a vertical minor axis with points: (x, y) ==> (4, 1) which has the equation below:

Substitute 4 for x1 and substitute 1 for y1

`((x-4)^2)/(a^2)+((y-1)^2)/(b^2)=1`

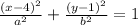
Where:

`c^2=a^2+b^2`

Since the minor axis is vertical and has a length of 8, we have:

`\begin{gathered} 2b=8 \\ \\ \text{Divide both sides by 2:} \\ (2b)/(2)=(8)/(2) \\ \\ b=4 \end{gathered}`

Given: c = 3

To find the value of a, substitute 3 for c and 4 for b:

`\begin{gathered} c^2=a^2+b^2^{} \\ \\ 3^2=a^2-4^2 \\ \\ \text{Add 4}^2\text{ to both sides:} \\ 3^2+4^2=a^2-4^2+4^2 \\ \\ 3^2+4^2=a^2 \\ \\ \text{Take the square root of both sides:} \\ \sqrt[]{3^2+4^2}=\sqrt[]{a^2} \\ \\ \sqrt[]{3^2+4^2}=a \\ \\ \sqrt[]{9+16}=a \\ \\ \sqrt[]{25}=a \\ \\ 5=a \\ \\ a=5 \end{gathered}`

Therefore, from the general equation, substitute 5 for a, and 4 for b

`\begin{gathered} ((x-4)^2)/(a^2)+((y-1)^2)/(b^2)=1 \\ \\ \\ ((x-4)^2)/(5^2)+((y-1)^2)/(4^2)=1 \\ \\ \frac{(x-4)^2}{25^{}}+\frac{(y-1)^2}{16^{}}=1 \end{gathered}`

Therefore, the equation for thr given ellipse that satisfies the given conditions is:

`\frac{(x-4)^2}{25^{}}+\frac{(y-1)^2}{16^{}}=1`

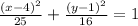
ANSWER:

`\frac{(x-4)^2}{25^{}}+\frac{(y-1)^2}{16^{}}=1`