Question

Which of the following is NOT a zero of the function f(x) = 2x^4 + x^3 – 5x^2 + 2x?1/2 0 -1 -2

Answer 1

Answer: 1/2, 0, and -2

Given:

`f(x)=2x^4+x^3-5x^2+2x`

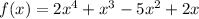
To know if a given value is a zero of a function, f(x) must be equal to 0. With this, we will substitute the given values and see which values will result in f(x)=0.

`\begin{gathered} f(x)=2x^4+x^3-5x^2+2x \\ f((1)/(2))=2((1)/(2))^4+((1)/(2))^3-5((1)/(2))^2^{}+2((1)/(2))=0 \\ f(0)=2(0)^4+(0)^3-5(0)^2+2(0)=0 \\ f(-1)=2(-1)^4+(-1)^3-5(-1)^2+2(-1)=-6 \\ f(-2)=2(-2)^4+(-2)^3-5(-2)^2+2(-2)=0 \end{gathered}`

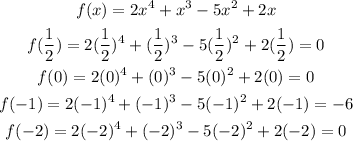
From these, we can see that the values 1/2, 0, and -2 resulted in f(x)=0. Therefore, the answers are 1/2, 0, and -2.