Question

What is the radius of the circle write your answer in simplified, rationalized form

Answer 1

`r=(√(37))/(2)`

Step-by-step explanation: We have to find the radius of the circle, the equation of the circle is as follows:

`x^2+y^2-3x-7=0\rightarrow(1)`

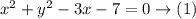
We will compare it with the standard equation of the circle, which is as follows:

`(x+a)^2+(y+b)^2=r^2\rightarrow(2)`

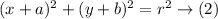
By inspecting equation (1) and comparing it with the equation (2) we can tell that b = 0, further on:

`\begin{gathered} x^2+-3x-7+y^2=0 \\ x^2+2ax+a^2+y^2-r^2=0 \\ \text{ Comparison gives the following:} \\ 2a=-3\rightarrow a=-(3)/(2)\rightarrow a^2=(9)/(4) \\ \therefore\rightarrow \\ x^2-3x+(9)/(4)-(37)/(4)+y^2=0 \\ (x-(3)/(2))^2+y^2=(37)/(4) \\ \therefore\rightarrow \\ r^2=(37)/(4) \\ r=(√(37))/(2) \end{gathered}`

The following graph confirms the answer: