Question

Cost and revenue are expressed in dollars. It have three parts.

Answer 1

a) 1502 units, 52570 dollars

b)

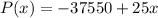`P(x)=-37550+25x`Step-by-step explanation

given

`\begin{gathered} C(x)=37550+10x \\ R(x)=35x \end{gathered}`

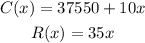
Revenue is equal to the number of units sold times the price per unit and

Total cost = Units sold * Variable cost per unit + Fixed costs

so

Step 1

The break-even point is the point at which total cost and total revenue are equal

a)to find the number of units that must be sold, we need set equalt the cost and revenue equations and solve for x, so

`\begin{gathered} C(x)=R(x) \\ 37550+10x=35x \\ subtract\text{ 10 x in both sides} \\ 37,550+10x-10x=35x-10x \\ 37550=25x \\ divide\text{ both sides by 25} \\ (37,550)/(25)=(25x)/(25) \\ 1502=x \end{gathered}`

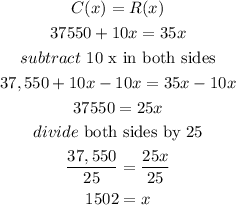
so, 1502 units must be sold

Step 2

now, to find the total dollar amount coming in and coming out we need to evaluate the x value we found in the previous step, so

`\begin{gathered} C(x)=37550+10x \\ let\text{ x= 1502 , replace and calculate} \\ C(1502)=37,550+10*1502 \\ C(1502)=52570 \\ and \\ R(x)=35x \\ let\text{ x= 1502} \\ replace\text{ and calculate} \\ R(1502)=35*1502 \\ R(1502)=52570 \end{gathered}`

therefore, the amount is 52570 dollars

Step 3

b)Profit function

profit functions is calculate as follows :

Profit function=the revenue function minus the cost function

so

`\begin{gathered} P(x)=35x-(37550+10x) \\ P(x)=35x-37750-10x \\ P(x)=-37550+25x \end{gathered}`

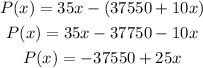
so, the profit function is

`P(x)=-37550+25x`

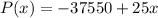
I hope this helps you