ANSWER:
(a)
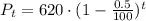
(b) 561 thousand people
(c) During the year 2074
Explanation:
(a) We can establish the formula to determine the population as follows:
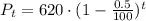
(b) To calculate the population, we know that the year 2030 would be t = 20 (2030-2010), therefore:
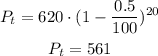
(c) In this case, we know that the value of Pt is equal to 450, we replace and solve for t, like this:
