We are told that the function that describes the position of the stone is given by the function
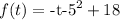
recall that the velocity is the derivative of the position. So we need to calculate the derivative. Recall that the derivative of a function of the form
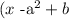
where a and b are constants, is
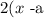
So, applying this, we get
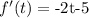
we want to find the value of this new function when t=6. So we have

so when t=6 we have the velocity is -2 m/s. This means that option B is correct.