Question

Solve b, I understand a and c so I included it for reference

Answer 1

Given:

`T=10*2^(-0.274a)`

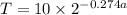
To Determine: The average rate of change of T with respect to a over the interval of 24000 and 30000

Solution

Let us determine the value of a at the interval given

`\begin{gathered} a=0\text{ correspond to 22000ft} \\ at\text{ 24000ft,} \\ a=(24000-22000)/(1000) \\ a=(2000)/(1000) \\ a=2 \\ at\text{ 30000ft} \\ a=(30000-22000)/(1000) \\ a=(8000)/(1000) \\ a=8 \end{gathered}`

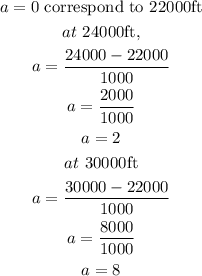
Let us determine the value of T at the given interval using the values of a

`\begin{gathered} a=2 \\ T=10*2^(-0.274(2)) \\ T=10*2^(-0.548) \\ T=10*0.683968 \\ T=6.83968 \end{gathered}`
`\begin{gathered} a=8 \\ T_8=10*2^(-0.274*8) \\ T_8=10*2^(-2.192) \\ T_8=10*0.218848 \\ T_8=2.18848 \end{gathered}`

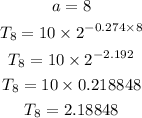
The rate of change would be

`\begin{gathered} r=(T_8-T_2)/(a_8-a_2) \\ r=(2.18488-6.83968)/(8-2) \\ r=-(4.6512)/(6) \\ r=-0.7752min-per-1000ft \\ r=-0.7752*60sec-per-1000ft \\ r=-46.512s-per-1000ft \end{gathered}`

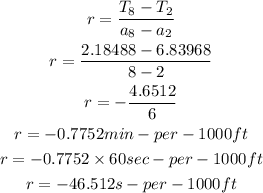
Hence, the rate of change of T with respect to a is -46.512 seconds per 1000ft