Question

A rain drop hitting a lake makes a circular ripple. If the radius, in inches, grows as a function of time in minutes according to r(t)=25t+2−−−−√ , find the area of the ripple as a function of time. Find the area of the ripple at t=2 .Enter the exact answer. For the number π , you can either type pi or you can click the button with π on it.Do not enter any commas in your answer.

Answer 1

INFORMATION:

We know that:

- A rain drop hitting a lake makes a circular ripple

- the radius, in inches, grows as a function of time in minutes according to r(t) = 25√(t+2)

And we must find the area of the ripple as a function of time and the area of the ripple at t=2

STEP BY STEP EXPLANATION:

To find it, we must:

1. Write the equation for the area of a circle

`A=\pi* r^2`

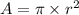
2. Write the given equation for the radius

`r(t)=25√(t+2)`

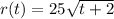
3. Replace the equation for the radius in the equation for the area of a circle

`A(t)=\pi*(25√(t+2))^2`

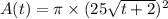
4. Find the area when t = 2 replacing it in the function for the area

`\begin{gathered} A(2)=\pi*(25√(2+2))^2 \\ \text{ Simplifying, } \\ A(2)=\pi*(25√(4))^2 \\ A(2)=\pi*50^2 \\ A(2)=2500\pi \end{gathered}`

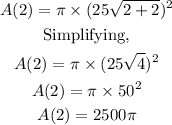
Finally, the area of the ripple at t=2 is 2500π in^2

ANSWER:

Area of the ripple as a function of time:

`A(t)=\pi*(25√(t+2))^2`

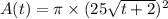
Area of the ripple at t=2:

A = 2500πin ^2