Since the actual length of Fence A is 1 1/5 yards
Since its length on the blueprint is 1 4/5 inches
Since the actual length of the Fence, B is 6 yards
Then we will use the ratio method to find its length in the blueprint
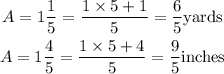
Then by using the ratio method
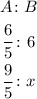
By using the cross multiplication
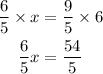
Divide both sides by 6/5 to find x
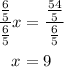
Fence B is 9 inches in the blueprint