Question

In a recent awards ceremony, the age of the winner for best actor was 38 and the age of the winner for best actress was 51. For all best actors, the mean age is 46.8 years and the standard deviation is 5.7 years. For all best actresses, the mean age is 31.9 years and the standard deviation is 10.8 years. (All ages are determined at the time of the awards ceremony.) Relative to their genders, who had the more extreme age when winning the award, the actor or the actress? Explain.

Answer 1

Given

the age of the winner for best actor was 38 and the age of the winner for best actress was 51.

For all best​ actors, the mean age is 46.8 years and the standard deviation is 5.7 years.

For all best​ actresses, the mean age is 31.9 years and the standard deviation is 10.8 years.​

Find

who had the more extreme age when winning the​ award, the actor or the​ actress

Step-by-step explanation

the Z- score is given by

`Z=(X-\mu)/(\sigma)`

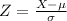
Best Actor

X = 38

mean = 46.8 years

standard deviation = 5.7 years.

so ,

`\begin{gathered} Z=(38-46.8)/(5.7) \\ \\ Z=-1.54385964912\approx-1.54 \end{gathered}`

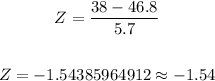
Best Actress

X = 51

mean = 31.9 years

standard deviation = 10.8 years.

so ,

`\begin{gathered} Z=(51-31.9)/(10.8) \\ \\ Z=1.76851851852\approx1.77 \end{gathered}`

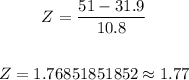
the best actress's age is farther from the mean so he has the more extreme age when the winning the award.

Final Answer

Hence , the z- score for best actor is -1.54 and for best actress = 1.77

Actress has more extreme age