Given:
a.) The admission fee at a small fair is $2.50 for children and $4.00 for adults.
b.) On a certain day, 2400 people enter the fair and $7140 is collected.
Let,
x = total number of children
y = total number of adults
Let's generate two equations based on the given scenario:
EQUATION 1: Total number of children and adults entered the fair.
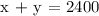
EQUATION 2: Total money collected from the admission.
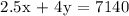
We will be using the substitution method. We get,
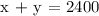

Substitute to Equation 2:
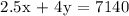
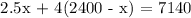
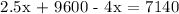
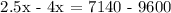

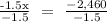
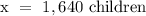
Therefore, 1,640 children went to the fair.
For the adults,
x + y = 2400
1,640 + y = 2400
y = 2400 - 1,640
y = 760 adults
In summary: 1,640 children and 760 adults went to the fair.