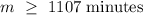Step 1: Concept
Given data
Monthly fee = $20
Additional cost per minute = $0.07
Let number of minutes = m
Step 2: Write an inequality equation that will represent the least cost in a month.
In mathematics, the word 'least' represents greater than or equal to.
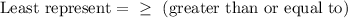
Step 3: Write an inequality equation.
For the least cos t of $97.49 per month.

Step 4: Final answer
The possible number of minutes is