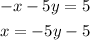Answer:
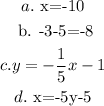
Explanation:
Solve the equations using inverse operations to solve equations. Remember that addition and subtraction are inverse operations, such as multiplication and division.
Therefore,
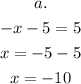
b. Substitute x=3 into the given expression.
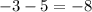
c. Isolate y in the given equation:
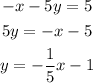
d. Isolate x in the given equation: