You have the following quadratic function:
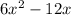
the previous function is a parabolla with a positive leading coefficient equal to 6 (leadding coefficient is the coefficient of the quadratic term). It means that the parabola opens up and then the parabolla has a minimum point.
The x-coordinate of the minimum point is just the x-coordinate of the vertex, which is given by:

a and b are coefficients of the quadratic function. In this case a=6 and b=-12.
Replace the previous parameters into the expression for x and simplify:
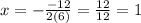
Now, the y-coordinate of the vertex (minimum point in this case) is:

Then, the minimum of the function is at the point (1 , -6)