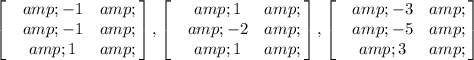Given:
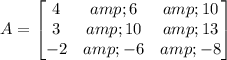
First we need to find the eigenvalues of A. Recall that they are the solutions of the equation det(λI - A) = 0:
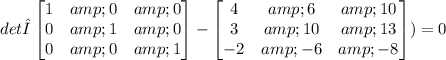
which becomes
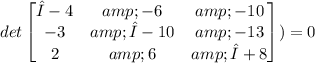
Calculate this determinant:
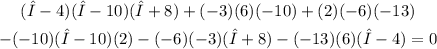
Simplify:
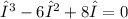
Then, factor:
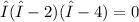
Separate the solutions:
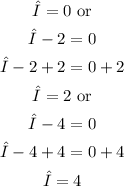
Now that we have found the eigenvalues for A , we can compute the eigenvectors:
For λ = 0

For λ = 2
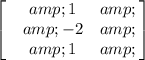
For λ = 4
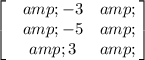
Answer:
The eigenvalues are:
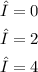
And the eigenvectors are: