Question

A certain kind of sheet metal has, on average, 5 defects per 11 square feet.Assuming a Poisson distribution, find the probability that a 19 square foot metal sheet has at least 9 defects. Round your answer to three decimal places.

Answer 1

Given:

The metal sheet has 5 defects per 11 square feet.

To find:

The probability that a 19 square foot metal sheet has at least 9 defects.

Step-by-step explanation:

On an average,

Then, using the poison distribution,

`\begin{gathered} P\left(X≥9\right)=1−P\left(X<9\right) \\ =1−e^(-λ)\sum_{i\mathop{=}0}^8(λ^i)/(i!) \\ =1−e^(-8.64)\sum_{i\mathop{=}0}^8(8.64^i)/(i!) \\ =1-0.5039 \\ =0.49606 \\ \approx0.496 \end{gathered}`

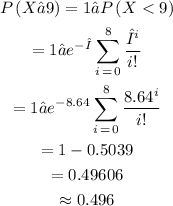
Thus, the probability that a 19 square foot metal sheet has at least 9 defects is 0.496.

Final answer:

The probability that a 19 square foot metal sheet has at least 9 defects is 0.496.