Question: Three ships, A, B, and C, are anchored in the Atlantic ocean. The distance from A to B is 36.318 miles, from B to C is 37.674 miles, and from C to A is 11.164 miles. Find the angle measurements of the triangle formed by the three ships.
Solution:
Note: In finding the angles of a triangle given its three sides, we will use the Cosine Law.
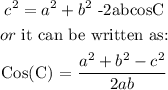
In finding angle C, we use the formula given above.
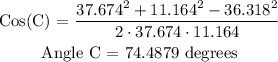
Note: Side a is the side opposite Angle A, side b is the side opposite Angle B, and side c is the side opposite Angle C.
Let's find the next angle.
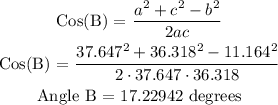
Note: We can still use the cosine law in finding Angle A. But another solution is subtracting the Angles A and B from 180 degrees. The measure of the internal angle of a triangle is always 180 degrees no matter what type of triangle it is.
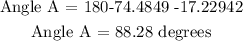
ANSWER:
A. m∠A=88.28267; m∠B=17.22942; m∠C=74.4879