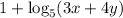Question

Use the properties of logarithms to expand the following expression as much as possible. Simplify any numerical expressions that can be evaluated without a calculatorlog5 (15x + 20y)

Answer 1

The given expression is

`\log _5(15x+20y)`

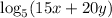
Factor out the greatest common factor between 15x and 20y.

`\log _5(5(3x+4y))`

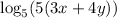
Then, use the product property to expand the logarithm.

`\log _55+\log _5(3x+4y)`

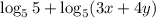
At last, simplify the first logarithm because its base is equal to its argument.

`1+\log _5(3x+4y)`

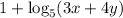
Therefore, the simplest form of the logarithm is

`1+\log _5(3x+4y)`