Question

A study determined that 9% of children under 18 years of age live with their father only. Find the probability that at most 2 persons selected at random from 12 children under18 years of age lived with their father onlyThe probability that at most 2 children live with their father only is(Do not round until the final answer. Then round to the nearest thousandth as needed)

Answer 1

Step 1: Write out the formula for binomial distribution

`P(x)=^nC_x* p^x* q^(n-x)`

Where

`\begin{gathered} p\Rightarrow\text{probability of success} \\ q\Rightarrow\text{probability of failure} \\ n\Rightarrow\text{ number of trails } \\ x\Rightarrow\text{ number of success required} \end{gathered}`

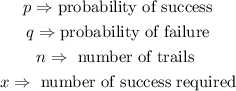
Step 2: State out the parameters needed in the formula to find the probabilty

`\begin{gathered} p=9\text{ \%=}(9)/(100)=0.09 \\ q=1-p=1-0.09=0.91 \\ n=12 \\ x\Rightarrow\le2\Rightarrow0,1,2 \end{gathered}`

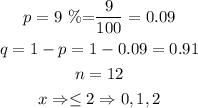
Step 3: The probability that at most 2 children live with their father only can be described as;

`P(x\le2)=P(0)+P(1)+P(2)`

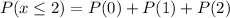
Step 4: Find the probability of each number of successes required

`\begin{gathered} P(0)=^(12)C_0*(0.09)^0*(0.91)^(12-0) \\ P(0)=1*1*0.322475487=0.322475487 \end{gathered}`
`\begin{gathered} P(1)=^(12)C_1*(0.09)^1*(0.91)^(12-1) \\ =^(12)C_1*(0.09)^1*(0.91)^(11) \\ =12*0.09*0.354368667=0.38271816 \end{gathered}`
`\begin{gathered} P(2)=^(12)C_2*(0.09)^2*(0.91)^(12-2) \\ =^(12)C_2*(0.09)^2*(0.91)^(10) \\ =66*0.0081*0.389416118=0.208181856 \end{gathered}`

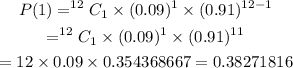
Step 5: Add all the number of successess required

`\begin{gathered} P(x\le2)=0.322475487+0.38271816+0.208181856 \\ =0.913375503 \\ \approx0.913 \end{gathered}`

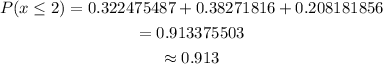
Hence, the probability that at most 2 children live with their father only is 0.913