a. The given table is
Notice, the value of x increases at equal intervals of 1
Also, the value of y increases at an equal interval of 3
This means for the y values the difference between consecutive terms is 3
Also, for the x values, the difference between consecutive terms is 1
Hence, the table represents a linear function
The general form of a linear function is

Where m is the slope
From the interval increase
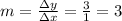
Hence, m = 3
The equation becomes
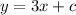
To get c, consider the values
x = 0 and y = 2
Thi implies
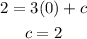
Hence, the equation of the linear function is

b. The given table is
Following the same procedure as in (a), it can be seen that there is no constant increase in the values of y
Hence, the function is not linear
This implies that the function is exponential
The general form of an exponential function is given as
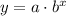
Consider the values
x =0, y = 3
Substitute x = 0, y = 3 into the equation
This gives

The equation become
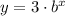
Consider the values
x =1, y = 6
Substitute x = 1, y = 6 into the equation
This gives
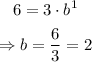
Therefore the equation of the exponential function is
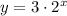
c. The given table is
As with (b) above,
The function is exponential
Using
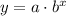
When
x = 0, y = 10
This implies

The equation becomes
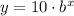
Also, when
x = 1, y =5
The equation becomes

Therefore, the equation of the exponential function is
