Step-by-step explanation
Let's see the facts:
-Morgan is making ----------------> 2 cookie recipes.
Recipe A ---> A = 2RecipeB -(1/3) 2RecipeB
-She needs-----------> Recipe A + Recipe B = 4 1/6 cups of sugar
Now, we have a system of equations:
(1) A = 2B -(1/3)2B
(2) A + B = 4 1/6
Multiplying both sides of (1) by 3:
3A = 6B - B
Simplifying:
3A = 5B
Isolating B:
B = 3/5 A
Substituting B-value in (2)
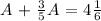
Reordering:
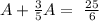
Multiplying both sides by 30:
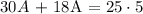
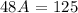
Dividing both sides by 48:
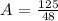
Representing as mix fraction and rounding:
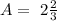
ANSWER: She will need two and two-thirds cups of Recipe A.