Given:
The objective is to find,
a) The probability of selecting a king or a queen.
b) The probability of selecting a face card or a 10.
Step-by-step explanation:
The total number of cards in a deck is, N = 52 cards.
a)
Out of 52 cards, the number of king cards is,
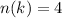
Similarly, out of 52 cards, the number of queen cards is,
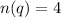
Then, the probability of drawing one out of 4 king cards or one out of 4 queen cards can be calculated as,
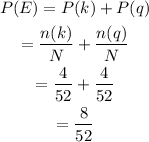
Hence, the probsability of selecting a king or a queen is (8/52).
b)
Out of 52 cards, the number of face cards is 12.
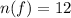
Similarly, out of 52 cards, the number of 10 is,
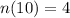
Then, the probability of drawing one out of 12 face cards or one out of 4 ten cards can be calculated as,
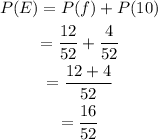
Hence, the probability of selecting a face card or a 10 is (16/52).
c)
Out of 52 cards, the number of spade cards is 13.
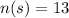
Similarly, out of 52 cards, the number of heart cards is 13.

Then, the probability of drawing one out of 13 spade cards or one out of 13 heart cards can be calculated as,
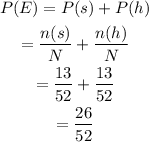
Hence, the probability of selecting a spade or a heart is 26/52.
d)
Out of 52 cards, the number of red cards is,
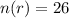
Out of 52 cards, the number of black cards is,
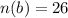
Then, the probability of drawing one out of 26 red cards or one out of 26 black cards is,

Hence, the probability of selecting a red card or a black card is 1.