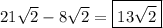Question

Add and subtract square roots that need simplification Number 186

Answer 1

Hello!

To solve this exercise, we must simplify these square roots until we have the same square root in both numbers (by the factorization process):

`3√(98)-√(128)`

First, let's factorize the square root of 98:

So, we know that:

`\begin{gathered} 3√(98)=3√(7^2*2)=3\sqrt[\cancel{2}]{7\cancel{^2}*2}=3*7√(2)=21√(2) \\ \\ 3√(98)=21√(2) \end{gathered}`

Now, let's do the same with the square root of 128:

So:

`√(128)=√(2^2*2^2*2^2*2)^1`

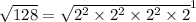
Notice that it also could be written as:

`\begin{gathered} √(128)=√(2*2*2*2*2*2*2) \\ \text{ or also} \\ √(128)=√(2^7) \end{gathered}`

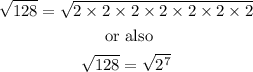
As we are talking about square roots, it will be easier if we group them in pairs of powers of 2, as I did:

`\sqrt[2]{128}=\sqrt[2]{2^2*2^2*2^2*2^1}`

Now, let's analyze it:

If the number inside the root has exponent 2, we can cancel this exponent and remove the number inside the root. Then, we can write it outside of the root, look:

`\begin{gathered} \sqrt[2]{128}=\sqrt[2]{2^{\cancel{2}}*2^{\cancel{2}}*2^{\cancel{2}}*2^1} \\ \sqrt[2]{128}=2*2*2\sqrt[2]{2^1} \\ \sqrt[2]{128}=8\sqrt[2]{2} \end{gathered}`

Now, let's go back to the exercise:

`\begin{gathered} 3√(98)-√(128)\text{ is the same as } \\ 21√(2)-8√(2) \end{gathered}`

So, we just have to solve it now:

`21√(2)-8√(2)=\boxed{13√(2)}`